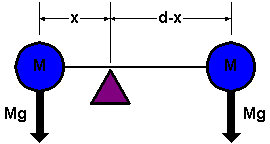Newton's Universal Law of Gravitation

Every particle in the Universe attracts every other particle with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.

This is Newton's Universal Law of Gravitation in words. In mathematical notation, let the two masses be m₁ and m₂, and let the distance between their centers be designated r. Then we write

Notice some features of this force. First, it is proportional to 1/r². We say that this is an inverse square law force, that is, the force is "inversely proportional to the square of the distance". If the distance between two objects is doubled, the force decreases by a factor of 4 (is 1/4 the original), or if the distance between two objects is halved, the force increases by a factor of 4.

Next, the force of m₁ on m₂, written F₂₁, is equal in magnitude to the force of m₂ on m₁, written F₁₂. The gravitational force is always attractive, so the directions of F₂₁ and F₁₂ are opposite. Written mathematically, F₂₁ = -F₁₂ as required by Newton's third law.

If one or both masses are extended spherical objects (the usual case for our work), then the same law holds, as long as the distance between the objects is measured between their centers, and the objects don't overlap. Pay attention to objects overlapping when, for instance, calculating what happens to something "launched" from the surface of the earth into space.

Example: P7.32

During a solar eclipse, the Moon, Earth, and Sun all lie on the same line, with the Moon between the Earth and the Sun. (a) What force is exerted on the Moon by the Sun? (b) What force is exerted on the Moon by the Earth? (c) What force is exerted on the Earth by the Sun?

From problem 31, the distance from the Earth to the Moon is 384,000 km = 3.84x10⁸ m, and from table 7.3, the distance from the Earth to the Sun is 1.496x10¹¹ m. During a solar eclipse, the distance from the Moon to the Sun is the difference of the above distances, or 1.492x10¹¹ m. The mass of the Sun is 1.991x10³⁰ kg, the Earth is 5.98x10²⁴ kg, and the Moon is 7.36x10²² kg.

(a) F_moon-sun = (6.673x10^-11 Nm²/kg²)(1.991x10³⁰ kg)(7.36x10²² kg)/(1.492x10¹¹ m)² = 4.39x10²⁰ N
(b) F_moon-earth = 1.99x10²⁰ N
(c) F_earth-sun = 3.55x10²² N

Historically, there was one small difficulty with the law of gravitation applied to planets and stars: for n gravitational relations, there were n+1 unknown quantities, namely G, plus n masses. This problem was finally solved in 1798 by Cavendish (112 years after Newton published his law in 1687). He arranged an apparatus to determine G, using known masses in his laboratory. See the text for a description.

Gravitational Potential Energy Revisited

In chapter 5 we introduced gravitational potential energy, and found that in a uniform gravitational field, like near the surface of the earth, PE = mgh. For objects far from the surface of the earth, this is no longer valid, and an alternate expression must be used:

PE = -GM_Em/r

M_E = 5.98x10²⁴ kg is the mass of the earth. This equation "fixes" the zero point of the potential energy at an infinite distance from the earth. And the above expression does reduce to mgh for objects near the surface of the earth, with g = GM_E/R_E² = (6.67x10^-11 N m²/kg²)(5.98x10²⁴ kg)/(6.38x10⁶ m)² = 9.84 m/s².

Example: Escape Speed from the Earth

Let's apply the general gravitational potenttial energy formula to determine the minimum speed necessary for an object to escape Earth's gravity. First, what is the meaning of "escape earth's gravity", given the fact that the gravitational force reaches to infinity? We will say that an object can escape earth's gravity if it has sufficient speed to (eventually) travel an infinite distance from Earth. When given the minimum speed, the object will arrive at infinity with exactly zero speed.

We will use conservation of mechanical energy. When the object reaches infinity with zero speed, its kinetic energy is zero, and at infinity, the gravitational potential energy is also zero, so the final mechanical energy is zero.

The initial mechanical energy is E_i = KE_i + PE_i = ½mv_i² - GM_Em/R_E. We use R_E, since the object starts at the surface of the earth, a distance R_E from the center.

Setting E_i = E_f = 0 gives:

½mv_i² - GM_Em/R_E
or
v_i = Sqrt{2GM_E/R_E} = Sqrt{2(6.67x10^-11 N m²/kg²)(5.98x10²⁴ kg)/(6.38x10⁶ m)} = 1.12x10⁴ m/s = 11.2 km/s = 40,000 km/h = 25,000 mi/h.

Kepler's Laws

Before Newton wrote down the law of gravitation, Johannes Kepler deduced three "laws" about the orbits of planets around the sun. The three laws are:

1. All planets move in elliptical orbits with the Sun at one of the focal points.
2. A line drawn from the Sun to any plant sweeps out equal areas in equal time intervals.
3. The square of the orbital period of any planet is proportional to the cube of the average distance from the planet to the Sun.

The last one is of most interest as regards homework problems. The statement transforms into the equation:

T² = (4p²/GM_s) r³
where T is the orbital period of the planet, M_s is the mass of the sun, and r is the average distance from the planet to the Sun. This relation can be applied to the period of a satellite orbiting the earth by replaceing the mass of the sun with the mass of the earth, and using the average distance from the satellite to the center of the earth.

Example: Low Orbit Objects

All objects (satellites, space shuttles, space capsules, and space stations) in low earth orbit circle the earth approximately once every 90 minutes. Why?

Objects in low earth orbit are only a few hundred kilometers above the surface. This distance is small compared to the radius of the earth (the distance from the center of the earth to the surface), 6380 km. Let's apply Kepler's third law, using r = 6380 km = 6.38x10⁶ m.
T = 2p Sqrt{R_e³/GM_E} = 2p Sqrt{(6.38x10⁶ m)³/(6.67x10^-11 N m²/kg²)(5.98x10²⁴kg)} = 5070 s = 84.5 min.
This period is independent of the mass of the object, and the exact shape of the orbit.

Chapter 8: Rotational Equilibrium and Rotational Dynamics

We will complete our discussion of rotational motion by investigating rotational dynamics -- how forces produce rotational motion -- and equilibrium for objects that can rotate. In the process, we will come across the concepts of torque, angular momentum, angular kinetic energy, and conservation of angular momentum.

Torque

Torque is the measure of the ability of an applied force to cause rotation. Torque is denoted by the greek letter tau, t, and is equal to the applied force times the "lever arm", d,
t = Fd.
The units of torque are force times distance, or Nm. (People may also be familiar with the English unit ft lb.) The lever arm is the perpendicular distance from the axis of rotation to a line drawn parallel to the force. Alternatively, we can take the lever arm as the distance from the axis of rotation to the point where the force is applied, but then we must use only the component of the force perpendicular to the lever arm.

Examples:

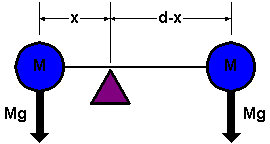Some examples to help understand how to calculate torque.

1. A wrench: See Fig. 8.2 in the text.
2. A door.
3. A barbell supported off center, as drawn to the right. The triangle represents the point of support. There are two torques in this example, one from the weight on the left which tends to rotate the barbell counterclockwise, and one from the weight on the right which tends to rotate it clockwise. As you might imagine, we assign torques that tend to cause a counterclockwise (CCW) rotation a positive value, and those that tend to cause a clockwise (CW) rotattion a negative value. Therefore, the torque from the left mass is t_left = +Mgx, and the torque from the right mass is t_right = -Mg(d-x). The total torque is a sum of the two, t_tot = t_left + t_right = Mgx - Mg(d-x) = Mg(2x-d). Notice that if the support is in the middle, x=d/2, then the total torque is zero. Also, note that the support must supply a normal force, n = 2Mg, which supports the whole barbell, but since this force acts at the point of support, it's torque is zero.