Two mirrors make an angle of 70° with each other.
If a light ray is incident at an angle of 20°, and reflects off both mirrors, what is the direction of the outgoing ray?Light is a wave. More precisely it is an electromagnetic wave. Light travels in vacuum at the "speed of light" which is given the symbol c = 2.99792458×108 m/s exactly. This number is no longer an experimental measurement but is now defined. And we use the speed of light and the measurement of time to define the length of the meter. One meter is the distance light travels in vacuum in (1/299792458) seconds.
When traveling in a medium other than vacuum, it travels at a speed less than the speed of light. For instance, in glass, the speed of light is about 2×108 m/s, or about 2/3 of the speed of light in vacuum.
Light has two possible polarizations. The directions of polarization are perpendicular to the direction of propagation, so if light is traveling the z direction, then the directions of polarization lie in the x-y plane. Polarized glasses block light components along one direction of polarization.
"Light travels in a straight line path until it encounters a boundary between two different materials." At the boundary, light either passes through, is reflected, or does a little of both.
Using the idea that light travels in straight lines, we will represent a beam of light by straight lines, so called rays. The rays are straight lines moving through a uniform medium. At a boundary, the lines can bend, as we will see. Because of the process of drawing straight lines and determining the angles between them, this approach to optics is called "geometric optics".
When a ray traveling through a medium strikes a boundary, part of it is reflected. The reflection can be either diffuse or specular. Diffuse reflection happens from a rough or irregular boundary -- an incoming beam of parallel rays are scattered at different angles, i.e. diffusely. Specular reflection occurs from a smooth polished surface and is the focus of our interest. In the following, "reflection" will mean "specular reflection".
When a beam of light reflects from a smooth surface, the scattered rays come out parallel to each other. The angle of the scattered rays equals the angle of the incoming rays. It is standard practice to measure these angles with respect to a line perpendicular to the surface. This relation is then written qi = qr where I use "i" for incident and "r" for reflected.
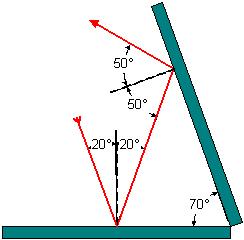
Two mirrors make an angle of 70° with each other.
If a light ray is incident at an angle of 20°, and reflects off both mirrors, what is the direction of the outgoing ray?
The angle of reflection equals the angle of incidence from the first surface, or 20° Extending the reflected ray to the second surface forms a triangle with the ray and the two mirror surfaces. The angle between the ray and the first surface is 90° - 20° = 70°, and the angle between the two mirror surfaces is 70°. Since the interior angles of a triangle must sum to 180°, the third angle (between the ray and the second surface) must be 180° - 70° - 70° = 40°. Therefore, the angle of incidence between the once reflected ray, and the second surface is 90° - 40° = 50°. And finally, the angle of the outgoing ray is equal to the incident angle of 50°.
When a light ray reaches a boundary part of the light can enter the medium.
The light entering the medium is bent, or refracted.
If we call the incident angle q1 and the refracted angle q2, then:
(sinq2)/(sinq1) = v2/v1
where v1 is the speed of light in medium 1 and v2 is the speed of light in medium 2.
This relationship is known as Snell's Law.
When light passes into a medium where the speed of light is lower, the ray is bent towards the perpendicular to the surface. When light passes into a medium where the speed of light is higher, the ray is bent away from the perpendicular.
It is traditional to work with a quantity called the index of refraction rather than the speed of light. The index of refraction, n, is defined as:
| n = | speed of light in vacuum | = | c |
| speed of light in a medium | v |
The index of refraction is a dimensionless number greater than 1. Table 22.1 in the text contains a list of indices of refraction for a number of substances.
Since, for two media, 1 and 2
v1 = fl1 and
v2 = fl2
for some frequency of light, f, we can write
(l1/l2) = (v1/v2) = (c/n1)/(c/n2) = n2/n1.
The ratio of wavelengths is the inverse of the ratio of the indices of refraction.
Or, written slightly differently:
l1n1 = l2n2.
Finally, we can use the index of refraction in Snell's Law.
From above we see that v2/v1 = n1/n2, and that we can therefore write:
n1sinq1 = n2q2.
This is the form of Snell's Law that is most useful.
The light beam shown in Figure P22.18 makes an angle of 20.0° with the normal line NN' in the linseed oil. Determine the angles q and q'. (The refractive index for linseed oil is 1.48.)
The refractive index of air is na » 1.00, of linseed oil is nl = 1.48, and of water is nw = 1.33.
Using Snell's Law
nasinq = nlsin 20.0°
for the air linseed oil interface, and
nwsinq' = nlsin 20.0°.
Solving for q and q' yields
sinq = (nl/na)sin 20.0° = (1.48/1)sin 20.0° = 0.506 or q = 30.4°
and
sinq' = (nl/nw)sin 20.0° = (1.48/1.33)sin 20.0° = 0.381 or q' = 22.4°.
Find the speeds of light in (a) flint glass, (b) water, and (c) zircon.
Use v = c/n, with c = 3.0×108m/s. (a) For flint glass, n = 1.66, and v = (3.0×108m/s)/1.66 = 1.81×108m/s. (b) For water n =1.33, and v = 2.26×108m/s. (c) For zircon n = 1.923, and v = 1.560×108m/s.
A light beam traveling in a medium with index of refraction n1 passes through a slab with parallel faces and index of refraction n2. Show that the emerging beam is parallel to the incident beam.
The fact that light passing through a flat slab comes out in the same direction that it went in at, is well known from looking through windows! To show this, we need to apply Snell's Law twice, once going from medium 1 to 2, and the second time going from 2 back to 1. Let's call the incident angle q1, the angle inside the slab q2, and the outgoing angle q3.
At the first interface, we have n2sinq2 = n1sinq1. At the second interface, the angle of the ray to the perpendicular to the surface is q2, thus we have n1sinq3 = n2sinq2. Inserting the first expression into the second we have n1sinq3 = n1sinq1, or sinq3 = sinq1, or more simply q3 = q1. Since the outgoing and incident angles are the same, we can see from the geometry that the beams are parallel.
The index of refraction is not necessarily the same for all wavelengths, in fact it normally varies with wavelength. This fact is called dispersion. Generally, the index of refraction is higher for shorter wavelengths, higher frequencies.
When complex optics are designed for cameras, binoculars, telescopes, or microscopes, care must be taken to minimize dispersion. The lenses are carefully chosen, and arranged to minimize the dispersion in the optics.
But dispersion is very useful when we do want to separate light into its component frequencies, for instance to see the spectral lines of the atoms and molecules in a substance.
Huygen's principle is a simple but powerful method to understand how light will move through certain complex systems. The basic idea is that a wave propagates due to every point on a given wavefront acting like a point source, producing outgoing spherical waves, the sum of which give the new wave front. It is difficult to sum all these spherical waves, luckily there is a simple method that works for an unobstructed wave: the new wavefront is the surface tangent to all the outgoing spherical waves.
For example, Figure 22.20 in the text shows an application of Huygen's principle to a plane wave and a spherical wave. Figure 22.21 is a picture of water waves in a ripple tank. Plane waves are incident from the bottom on a barrier with two slits in it. The slits act as point sources for outgoing spherical (circular) waves. The optical analog of this, called double slit interference, is discussed in Chapter 24.
Huygen's principle can be used to derive the equations for reflection and refraction. This is done in the text, I will omit the derivation in the interest of time.
Finally, we will look at an interesting phenomenon called total internal reflection. Total internal reflection can occur when light tries to pass from one medium to another with a lower index of refraction. For incident angles greater than a certain angle, called the critical angle, the light cannot exit and instead completely reflects back into the first medium. [See Fig. 22.24]
Let's look at Snell's Law, n1sinq1 = n2sinq2.
We want light to start in medium 1, and pass into medium 2 with a lower index of refraction, therefore n1>n2.
So we can write
sinq1 = (n2/n1)sinq2,
with n2/n1<0.
The maximum value of sinq2 is of course 1.0, and occurs when q2 = 90°.
For this angle of refraction, sinq1 = n2/n1 < 1.
We call this angle the critical angle, qc = Asin(n2/n1).
For incident angles greater than qc, we find that sinq1 must be greater than 1.0. This is not possible, agreeing with the observation that there is no refracted light, all of it is reflected from the boundary back into medium 1.
Total internal reflection is useful as a "perfect" reflector for a limited range of angles. And optical fibers rely on this principle to "trap" light inside the fiber and carry signals over many hundreds of kilometers.
A jewel thief hides a diamond by placing it on the bottom of a public swimming pool. He places a circular raft on the surface of the water directly above and centered on the diamond as shown in Figure P22.37. If the surface of the water is calm and the pool is 2.00m deep, find the minimum diameter of the raft that would prevent the diamond from being seen.
First of all, to "see" something means that it must be possible for a light ray to travel from the object to the viewer. For a light ray to travel from the diamond to a viewer outside of the water, it must pass from the water to the air. And this can only happen if the incident angle on the water-air boundary is less than the critical angle.
The index of refraction of air is 1.000, and of water is 1.333. The critical angle at the water-air boundary is qc = Asin(1.000/1.333) = 48.6°. To effectively hide the diamond, the raft must cover all angles less than 48.6°. Since the diamond is 2.00m below the surface and the raft, the radius of the raft must be r = (2.00m)tan 48.6° = 2.27 m. So the diameter of the raft must be d = 2r = 4.54m.