PHY5200 F07

Chapter 5: Oscillations

Reading

Taylor 5.2-5.4 (today), and 5.4-5.6 (Monday).

The second exam is Friday. There is also an SPS event on Friday.

On next homework assignment, any answer without explanation will lose half credit automatically. You MUST write some sentences on the page!

Points will be taken off for the use of vector quantities that lack any indication of the vector nature of the quantities .

Recall

X(t) = C₁e^iωt + C₂e^-iωt

x(t) = B₁cos(ωt) + B₂sin(ωt)

x(t) = A cos(ωt - δ)

x(t) = Re( Ce^iωt ) = Re( Ae^{i(ωt - δ)} )

Energy Considerations

Now let's look at the energy of the harmonic oscillator. Take the solution x(t) = A cos(ωt - δ) and substitute into the expression for the potential energy,

U = ½kx² = ½kA²cos²(ωt - δ)

Differentiate x(t) to obtain the velocity and use this to write the kinetic energy

T = ½mv² = ½kA²sin²(ωt - δ)

where we've used the relation ω=k/m. The total energy is the sum

E = T + U = ½kA²

a constant as it should be since the linear restoring force is conservative.

Two-Dimensional Oscillators

Isotropic Oscillator

D_t² x = -ω²x

D_t² y = -ω²y

x(t) = A_x cos(ωt - δ_x)

y(t) = A_y cos(ωt - δ_y)

where there are four constants to be determined by the initial conditions: A_x, A_y, δ_x, and δ_y. Four are required in general for two independent second order differential equaitons.

Anisotropic Oscillator

D_t² x = -ω_x²x

D_t² y = -ω_y²y

x(t) = A_x cos(ω_xt - δ_x)

y(t) = A_y cos(ω_yt - δ_y)

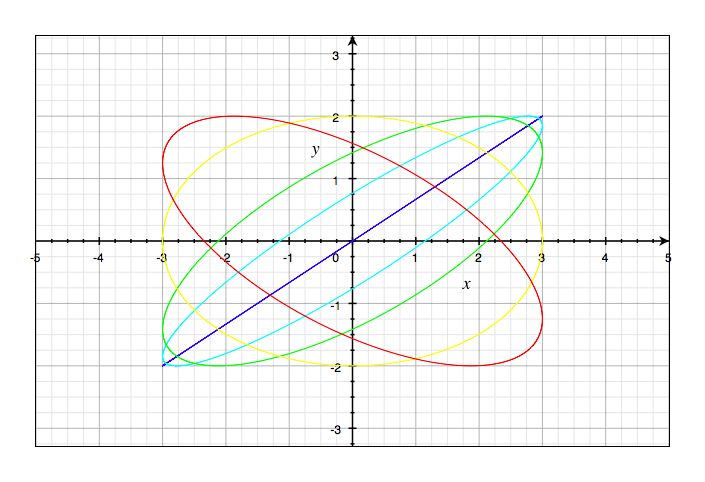
If ω_x/ω_y is a rational number, then the motion is periodic (i.e. the mass will eventually return to its initial position with its initial velocity and completely retrace the path it took to get there. If the ratio is irrational, then the motion never repeats.